Analysis of Transport Phenomena
Graduate-level introduction to mathematical modeling
Graduate-level introduction to mathematical modeling
Graduate-level introduction to mathematical modeling
In these MOOCs, you will learn to formulate mathematical models of transport phenomena based on partial differential equations and to solve them by pencil and paper. You will also learn the art of approximation—how to obtain useful solutions by simplifying a model without sacrificing the key physics. Applications include heat and mass transfer, fluid flow, waves, hydrodynamic instabilities, convection, phase transformations and electrochemical transport.
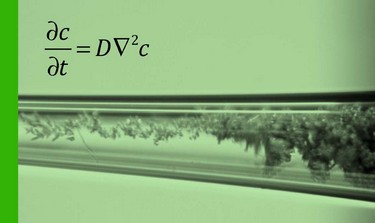

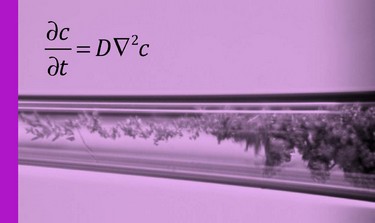
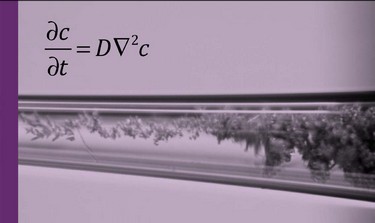
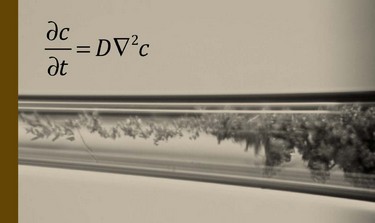
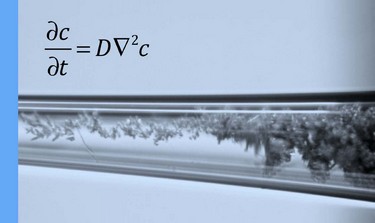
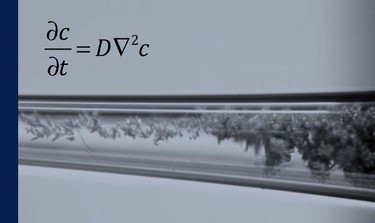

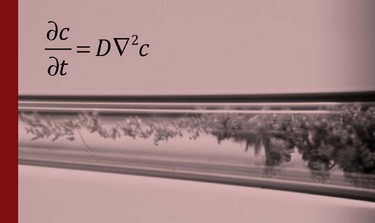
Models:
Mathematical Formulation:
Scaling:
Asymptotics:
Series Expansions:
Fluid Mechanics:
Convection:
Nonequilibrium Thermodynamics:
Electrochemical Transport:

